Saxon Math Is Going Away—What’s Next?

Posted on by

Written by Dr. James Carlovsky

With many WELS schools using Saxon Math as their curriculum, this recent information may have come like a punch in the gut. “Well . . . I’m contacting you on behalf of Houghton Mifflin Harcourt regarding your interest in Saxon Math. I wanted to let you know that the program will be fully retired and no longer available after 6/20/24.”

I’d like to share with you some essential philosophies to keep in mind, some common curricular alternatives, and some valuable resources for your classroom. In full transparency, most curricula have very similar scopes and sequences. So, what has the most significant impact on student learning? Teachers crafting quality lessons? Peers influencing understanding in a culture of discussion? Individual students who show grit? Curricula that give students meaningful experiences?

Before we get into common math philosophies, I have a few questions for your everyday math instruction:

  • How do you expect your students to show that they genuinely get it?
  • How do you know when your students are honestly thinking and learning?
  • Where do you find the time to improve your mathematics instruction if your work isn’t quite working?

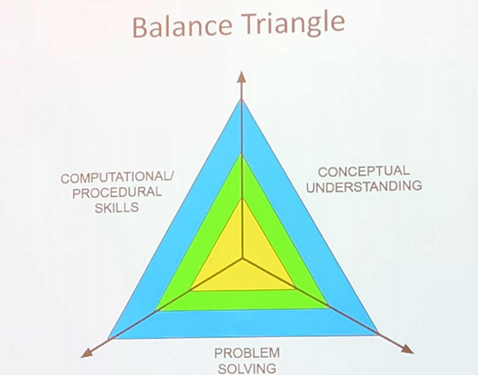I’d like to drive your attention to two models that have helped me as I’ve grown from my Saxon math roots as a student and teacher into a professor of future educators. The first is Jo Boaler’s (2015) Balance Triangle. Much attention has been given to computational skill building in many curricula like Saxon Math. This is driven by our society’s philosophy: “The faster you can compute, the better you are at math.” Notice that this triangle is a push to look at your instruction and find ways to give deeper understandings and real-life problem solving an important place within the curriculum.

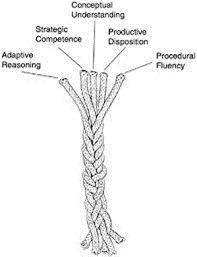Another similar model is the Five Strands of Math Proficiency. In 2001, the National Research Council sought to address a concern expressed by many Americans: that too few students in our schools are successfully acquiring the mathematical knowledge, skill, and confidence they need to use the mathematics they have learned. This model shows that these different portions must work together to help grow our students.

Before discussing common curricular choices, I’d like to recommend getting your students to talk! Stockero et al. (2011) found benefits to having students regularly share their mathematical thinking out loud in class, which can also increase student engagement and confidence. There are many student-centered ways get your students to do this:

  • Students show how to solve through small group explanations
  • Supporting students’ learning process through guidance
  • Students provide alternative ideas or multiple methods
  • Students clarify ideas and correct misconceptions
  • Students compare ideas with each other
  • Students question one another

No matter what curriculum you choose, how do you expect your students to show that they genuinely get it? What follows are some best practices to use in your classroom to prompt discussion.

  • Provide Support
    • “Is this like another problem?”
    • “Did you make a picture?”
    • “What is hard about this problem?”
    • “What have you tried?”
    • “Where did you get stuck?”
    • “How can you decide if this answer is correct?”
    • “What do you think is the right answer? Why?”
  • Find Out What Your Students Are Thinking
    • “Tell me about . . .”
    • “Why did you . . .”
    • “How did you . . .”
    • “What would happen if you . . .”
  • Provide Extensions
    • “Can you find another way?”
    • “What if you . . .?”
    • “Will it work if . . .?”
  • Post-Activity
    • “How did you solve this? What steps did you take?”
    • “Will your method always work?”
    • “Why did you do this?”
    • “How does this picture/model connect to your work?”
    • “What did you do that helped you understand the problem?”
    • “Was there something in the problem that reminded you of another problem?”
    • “Did you find information that you didn’t need? How did you decide?”
    • “How did you decide that your answer was correct?”
    • “Did you try something that didn’t work? How did you know?”
    • “Can something in this problem help you solve other problems?”

It is very difficult to know what exact textbook options may work best for your individual school’s needs. An excellent place to start is EdReports, which has thoroughly vetted many curricula. In my opinion, there are several reasons to ponder a blended curriculum:

Some schools have asked what other WELS schools are using. Here’s a partial list.

  • Horizons Math (spiral-bound like Saxon, geared for homeschooling or small school)
  • Eureka Math (deeper understanding for students, a learning curve for faculty, usually loved by year 3)
  • Bridges in Math (PK-gr 5, training required, investigations and resources)
  • Sadlier Math (traditional, looks like other bigger publisher books, side resources available)
  • Math in Focus (Singapore Math)

No matter what textbook series you choose, you can supplement to better accomplish your curricular goals.

Here are a few other resources you can probably use.

God’s blessings on your preparations and curriculum planning.

Dr. James Carlovsky (’02, MS Ed ’10) serves as a professor of mathematics and instructional technology at Martin Luther College.

References
Beckmann, S. (2014). Mathematics for elementary teachers with activities. Boston, MA: Pearson Education.

Boaler, J. (2015). Mathematical mindsets: Unleashing students’ potential through creative math, inspiring messages, and innovative teaching. John Wiley & Sons.

Carlovsky, J. (2022) Saxon Math No Longer Publishes: Now What Do We Do? [Google Slides Presentation]. Wisconsin State Lutheran Teachers’ Conference, 11 April, Milwaukee.

Humphreys, C., & Parker, R. (2015). Making number talks matter: Developing mathematical practices and deepening understanding, grades 4-10. Stenhouse Publishers.

Stockero, S. L., & Van Zoest, L. R. (2011). Connecting Research to Teaching: Making Student Thinking Public. The Mathematics Teacher, 104(9), 704-709. https://www.jstor.org/stable/20876997

 

 

Please, share YOUR thoughts!